
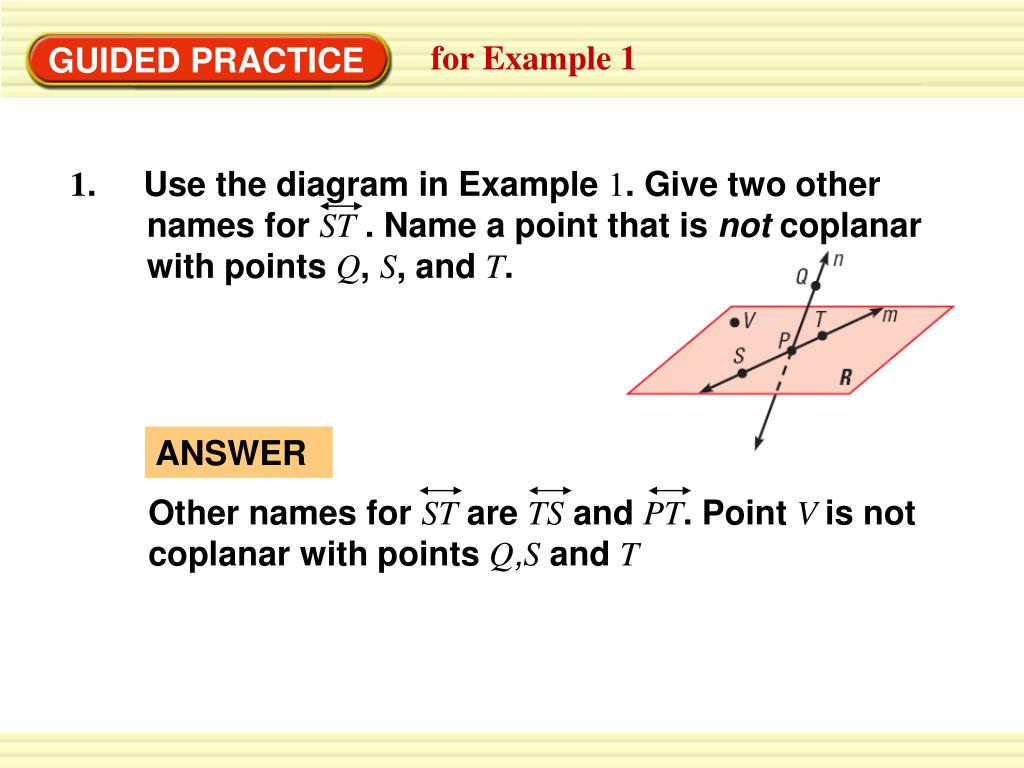
\(x \cdot = (2)\cdot – (0)\cdot + (3)\cdot\).Īs the triple product is non-zero, x, y & z are not coplanar. ‘a point which is not coplanar with the other two’ ‘Unfortunately, the researchers were unaware of a more overriding and crucial restriction - that the chemical groups on either side of the peptide bond were very likely to be coplanar. Applications of Coplanar VectorsĬoplanar vector is a mathematical structure and are widely used in the fields of Mathematics, Physics, Engineering Etc.
In the case of \(n\) vectors, if the coefficient’s determinant is zero or the coefficient’s matrix is a singular matrix, it is called a non-trivial solution.Īnd if the equation system has a determinant of the coefficient as a non-zero, yet the solutions are \(x=y=z=0\), then it is known as a trivial solution. No more than two vectors of ‘n’ are linearly independent then all vectors are coplanar.They are linearly independent then, the three vectors are coplanar.forces Coplanar non-concurrent forces Non-coplanar parallel forces Non-coplanar.
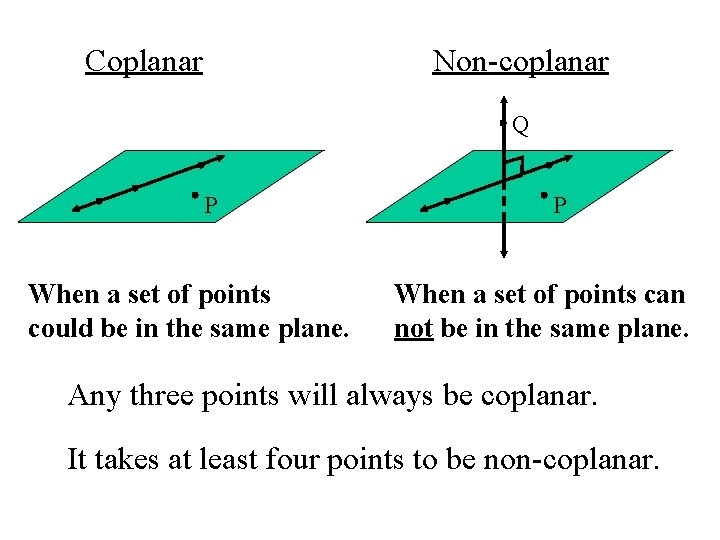
We establish an improved upper bound for the number of incidences. If all the forces in a system do not lie in a single plane they. But more than 3 points are usually NOT on the one. Incidences between points and non-coplanar circles. The triple scalar product is zero then, those three vectors are coplanar. 3 points are always coplanar because you can have a plane that they are all on. To determine whether the three vectors are coplanar or not, we often find the scalar triple product of the three vectors.


 0 kommentar(er)
0 kommentar(er)
